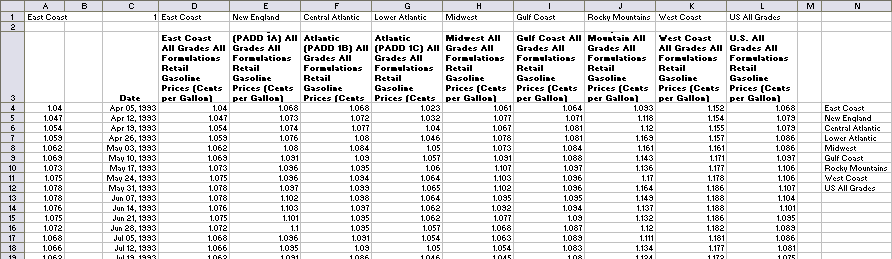
A college science professor named Roger Blickensderfer wrote to me about an animation he was working on. He wanted to trace the path followed by a point on the rim of a rolling wheel. Roger had cobbled something together, and I fiddled with it, and it kind of worked. But I decided I wanted it to do a bit more. I started with Roger’s basic idea and added my flexible animation from the Gas Prices – Animated Bar Chart for Excel example. I added a second point, which can be placed at a different distance from the axis of the wheel, and I added a checkbox to show or hide the curves traced by these two points.
I built an animation which can be extracted from this downloadable zip file. The top of the worksheet has the playback controls and other inputs to the left and the chart to the right. The first example uses a wheel radius of 10 and a second point at a radius of 0 (the axis of the wheel)
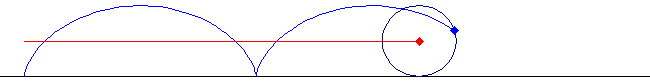
Press the button to start the animation. The red line stays at the same height, while the blue line traces a curve called a cycloid.



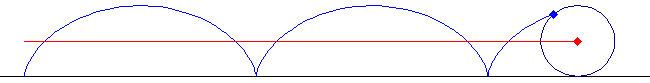
Here are the traces when the second point is at a radius of 5 (midradius).
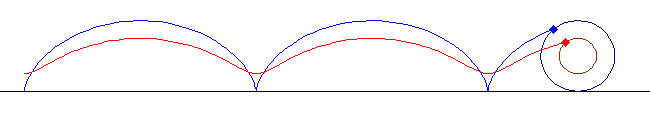
Here are the traces when the second point is at a radius of 15 (outside the radius of the wheel rim). This is similar to the case of a train wheel, which has a flange to keep the tain on the track. The outer surface of the flange actually moves backwards at the bottom of its travel.
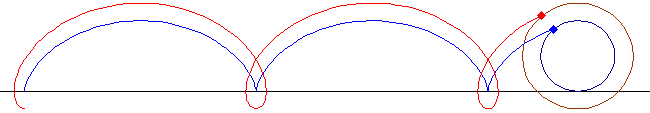
In this example, the second point has a radius of -10, so it appears on the rim opposite the first point.

I found another explanation of this in Rolling: the physics of wheels, on the web site of the School of Physics at The University of New South Wales. There is a large collection of similar animations on their Physclips page.